Sign up to get Today’s Best Articles in your inbox
Join 350,000+ subscribers and keep yourself informed with a selection of
The Week’s most interesting, enlightening and entertaining stories - plus daily puzzles.
You are now subscribed
Your newsletter sign-up was successful
Want to add more newsletters?
An account already exists for this email address, please log in.
How triangles revolutionized mapmaking
And more lessons from Ian Stewart's In Pursuit of the Unknown: 17 Equations That Changed the World
Equations are the lifeblood of mathematics, science, and technology. Without them, our world would not exist in its present form. However, equations have a reputation for being scary: Stephen Hawking's publishers told him that every equation would halve the sales of A Brief History of Time. [In Pursuit of the Unknown: 17 Equations That Changed the World]
Join more than 350,000 subscribers and keep yourself informed with a selection of The Week’s most interesting, enlightening and entertaining stories - plus daily puzzles.
Join more than 350,000 subscribers and keep yourself informed with a selection of The Week’s most interesting, enlightening and entertaining stories - plus daily puzzles.
There are two kinds of equations in mathematics. Stewart writes:
One kind presents relations between various mathematical quantities: the task is to prove the equation is true. The other kind provides information about an unknown quantity, and the mathematician's task is to solve it — to make the unknown known. The distinction is not clear-cut, because sometimes the same equation can be used in both ways, but it's a useful guideline. [In Pursuit of the Unknown: 17 Equations That Changed the World]
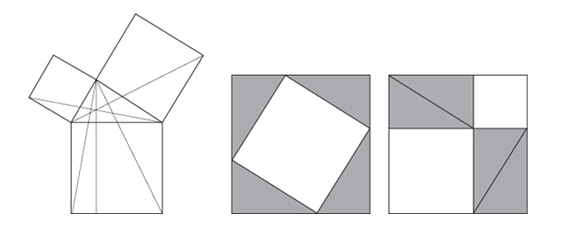
An example of the first kind of equation is Pythagoras' theorem, which is "an equation expressed in the language of geometry." If you accepts Euclid's basic geometric assumptions, then Pythagoras' theorem must be true.
Sign up to get Today’s Best Articles in your inbox
A free daily email with the day’s biggest news and analysis – and the best features from TheWeek.com
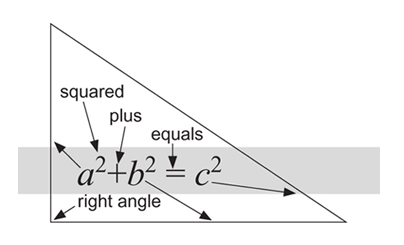
In right-angled triangles the square on the side subtending the right angle is equal to the squares on the sides containing the right angle.
Many triangles in real life are not right angled. But this does not limit the use of the equation a² + b² = c² because any triangle can be cut into two right-angled ones.
A good example of the second kind of equation is Newton's law of gravity. "It tells us how the attractive force between two bodies depends on their masses," Stewart writes, "and how far apart they are. Solving the resulting equations tells us how the planets orbit the Sun, or how to design a trajectory for a space probe." This isn't a mathematical theorem. Rather, it's true for physical reasons and fits our observations.
Equations, as simple as they appear, have redirected human history time and time again. "An equation derives its power from a simple source," Stewart writes. "It tells us that two calculations, which appear different, have the same answer."
The power of equations lies in the philosophically difficult correspondence between mathematics, a collective creation of human minds, and an external physical reality. Equations model deep patterns in the outside world. By learning to value equations, and to read the stories they tell, we can uncover vital features of the world around us. [In Pursuit of the Unknown: 17 Equations That Changed the World]
Pythagoras' theorem
This is the first equation presented in the book. As Stewart writes, it is not only "a vital link between geometry and algebra, allowing us to calculate distances in terms of coordinates. It also inspired trigonometry." What else did it inspire? "Surveying, navigation, and more recently special and general relativity — the best current theories of space, time, and gravity."
Think about it this way: Triangulation is an indirect way of determining distance by employing angles. Here's Stewart:
When surveying a stretch of land, be it a building site or a country, the main practical consideration is that it is much easier to measure angles than it is to measure distances. Triangulation lets us measure a few distances and lots of angles; then everything else follows from the trigonometric equations. The method begins by setting out one line between two points, called the baseline, and measuring its length directly to very high accuracy. Then we choose a prominent point in the landscape that is visible from both ends of the baseline, and measure the angle from the baseline to that point, at both ends of the baseline. Now we have a triangle, and we know one side of it and two angles, which fix its shape and size. We can then use trigonometry to work out the other two sides.
In effect, we now have two more baselines: the newly calculated sides of the triangle. From those, we can measure angles to other, more distant points. Continue this process to create a network of triangles that covers the area being surveyed. Within each triangle, observe the angles to all noteworthy features — church towers, crossroads, and so on. The same trigonometric trick pinpoints their precise locations. As a final twist, the accuracy of the entire survey can be checked by measuring one of the final sides directly. [In Pursuit of the Unknown: 17 Equations That Changed the World]
Surveys routinely employed triangulation by the late 18th century. And while we don't explicitly use it today, it is still there in how we deduce locations from satellite data.
Shane Parrish is a Canadian writer, blogger, and coffee lover living in Ottawa, Ontario. He is known for his blog, Farnam Street, which features writing on decision making, culture, and other subjects.
